What is Algebra?
Basic algebra is the field of mathematics that it one step more abstract than arithmetic.
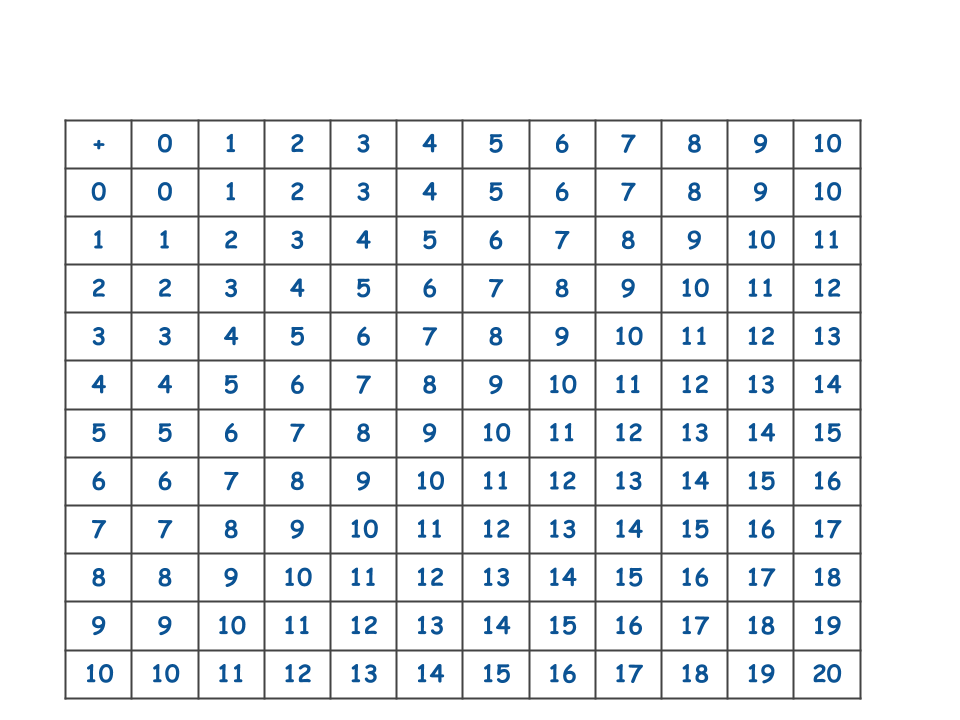
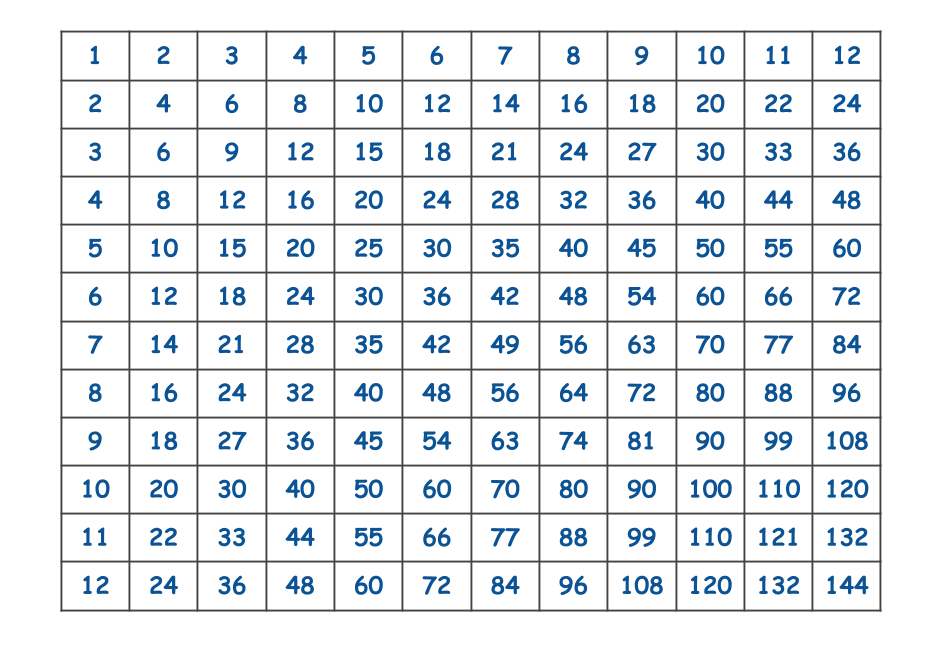
Algebra is one of the broad parts of mathematics, together with number theory, geometry and analysis. It uses variables to represent a value that is not yet known. When an equals sign (=) is used, this is called an equation. A very simple equation using a variable is: 2 + 3 = x. In this example, x = 5, or it could also be said that “x equals five”. This is called solving for x.
In its most general form, algebra is the study of mathematical symbols and the rules for manipulating these symbols, it is a unifying thread of almost all of mathematics.
It includes everything from elementary equation solving to the study of abstractions such as groups, rings, and fields.
The more basic parts of algebra are called elementary algebra; the more abstract parts are called abstract algebra or modern algebra.
Elementary algebra is generally considered to be essential for any study of mathematics, science, or engineering, as well as such applications as medicine and economics.
Abstract algebra is a major area in advanced mathematics, studied primarily by professional mathematicians.
Elementary algebra
Elementary algebra differs from arithmetic in the use of abstractions, such as using letters to stand for numbers that are either unknown or allowed to take on many values.
For example, in x+2=5 the letter x is unknown, but applying additive inverses can reveal its value: x=3.
In E=mc2, the letters E and m are variables, and the letter c is a constant, the speed of light in a vacuum. Algebra gives methods for writing formulas and solving equations that are much clearer and easier than the older method of writing everything out in words.
Advanced Algebra
Advanced algebra is the intermediate level Algebra. This algebra includes a high level of equations to resolve as compared to pre-algebra. Advanced algebra will help you to go through the other parts of algebra such as:
- Matrices
- Equations with inequalities
- Solving system of linear equations
- Sequences and series
- Graphing of functions and linear equations
- Polynomial Equation
- Trigonometry
- Rational expressions
- Polynomials and expressions with radicals
- Quadratic Functions with inequalities
- Discrete mathematics and probability
- Conic sections
Abstract Algebra
Abstract algebra deals with algebraic structures like the fields, groups, modules, rings, lattices, vector spaces, etc.
The concepts of the abstract algebra are:
- Sets – Sets is defined as the collection of the objects that are determined by some specific property for a set.
For example,
A set of all the 2×2 matrices, the set of two-dimensional vectors present in the plane and completely different form of finite groups. - Binary Operations – When the concept of addition is conceptualized, it gives the binary operations. The concept of all the binary operations will be meaningless without a set.
- Identity Element – The numbers 0 and 1 are conceptualized to give the idea of an identity element for a specific operation. Here, 0 is called the identity element for the addition operation, whereas 1 is called the identity element for the multiplication operation.
- Inverse Elements – The idea of Inverse parts comes up with a negative number. For addition, we write “-a” as the inverse of “a” and for the multiplication, the inverse form is written as “a-1″.
- Associativity – When integers are added, there is a property known as associativity in which the grouping up of numbers added does not affect the sum. An example, (3 + 2) + 4 = 3 + (2 + 4).
Linear Algebra
Linear algebra is a branch of algebra that applies to each applied as well as pure mathematics. It deals with the linear mappings between the vector spaces. It also deals with the study of planes and lines.
The important topics covered in linear algebra are as follows:
- Linear equations
- Relations
- Vector Spaces
- Matrices and matrix decomposition
- Relations and Computations
Commutative algebra
Commutative algebra is the branch of algebra that studies commutative rings, their ideals, and modules over such rings. The algebraic geometry and algebraic number theory build on commutative algebra. It examples of commutative rings include polynomial rings; rings of algebraic integers and so on.
Commutative algebra is the main technical tool in the local study of schemes.
The word algebra is also used in certain specialized ways. A special kind of mathematical object in abstract algebra is called an “algebra”, and the word is used, for example, in the phrases linear algebra and algebraic topology.
A mathematician who does research in algebra is called an algebraist.